Ev Formula Poker
Push ev =%of time you win. prize pool equity for winning + whatever prize you've already won. Fold ev = prize pool equity for folding. If push ev fold ev, ICMIZER tells you to push. Its actually not complicated math once you understand how to calculate it. Simple Poker EV Calculator EV, short for ‘expected value’ is the focus of every poker player. Our goal is to make as many +EV decisions as possible, and the more +EV the better. In short, a play that is +EV is expected to net us money over the long term while plays that are -EV are going to cost us money over the long term.
Before you get scared that this poker lesson is all about complex math, it’s not. Yes we’ll cover some of the calculations used to determine expected value in some common situations, but EV is just as much about a mindset as it is math. Instead of just thinking “I’m ahead” or “I’m behind“, the EV mindset is about putting your opponent(s) on a range of possible hands and developing a habit of quickly analyzing each possible decision and picking the one that will make you the most money in the long run.
As we take your understanding of expected value to another level, there are additional criteria that must be incorporated that will affect the long-term profitability of your decisions. The following are the primary criteria you will use in thinking about expected value:
- What is my opponents likely hand range?
- How likely is my opponent to fold if I bet?
- How likely is my opponent going to bet in this situation?
- How likely is my opponent bluffing in this situation?
- How likely is my opponent value betting in this situation?
- How likely will my opponent call with a hand I’m beating?
- How likely will my opponent fold a better hand if I bet?
- What will my stack size be as a result of my decision?
- What are the odds of improving my hand?
- What are my implied odds if I hit the card I am looking for?
Common EV Situations
One of the most common tournament poker scenarios where knowledge of expected value comes into play is when you’re short stacked and are faced with a decision to shove all-in or fold. Calculating your fold equity and the pre-flop ranges of your opponents is crucial. Put simply, the factors that will dictate your decision include:
- How much you win in blinds and antes if you’re not called
- How often you’re going to get called
- How wide your opponents calling range is
- Your hand and how it fares against your opponents range
- How much you gain if you get called and win
- How much you lose if you get called and lose
Your table image, your opponents’ images, your stack size and the stack sizes of the players left to act will play a big role in your opponents calling range. A very large stack size might decide to gamble more to bust you out, while a very short stack might feel desperate to double up; both of which may have a larger calling range. Of course a looser player will have a wider calling range, where a tight player may only call with premium hands.
Hand Example – Playing Against a Polarized Range
Let’s look at a few different hand examples and show how utilizing an understanding of expected value will help you earn more profit over the long run. This first hand is from a no-limit cash game with blinds of $0.50 and $1.00. Both you and your opponent started the hand with around 100 big blinds.
Pre-Flop / the Flop
It folds around to your opponent in the cut-off and he makes a standard 3x raise. Your opponent has been betting with a wide variety of hands and is a very aggressive player. You’re on the button with and while there are various ways to play your hand you decide to flat call in position. The blinds both fold and the flop comes . Your opponent makes a standard continuation bet of $4.50, just over half the pot.
Figure 1
You have middle pair on an Ace high board that’s quite co-ordinated. You also have the which is definitely good as it gives you some backdoor outs in case your opponent has an Ace. Although there are times when he could have an Ace, it’s more than likely that he’s continuation betting with a wide variety of hands since he’s a very aggressive player. Although in this situation there isn’t much value in raising because there is still a good chance he has an Ace, in which case he’d call any raise. So you decide to flat call and use the advantage of position.
The Turn & River
The turn card brings the and your opponent checks. He could be afraid of us having KJ but maybe he’s just pot controlling with a weak Ace, perhaps fearful that we also have an Ace with a stronger kicker. With him checking we can be fairly confident that he doesn’t have a huge hand like AA, KK or QT – since it’s more likely he’d choose to bet for value and want to charge us for possibly chasing a flush draw. So his range is fairly polarized from Aces to bluffs so far in this hand, and more than likely semi-bluffs. You decide to check and the river is which leads your opponent to push out a bet of $8 into the $16 pot.
Figure 2
Now you have to ask yourself… do I call? Am I getting the right pot odds and the equity versus his range of hands to make this a profitable call in the long run? There are definitely going to be times when he shows up with AT or A9 and is betting for value – in which case you can look somewhat stupid if you call and he does have an Ace. But remember, he is an aggressive opponent who could just be trying to get you to fold – perhaps a busted flush draw. So he has a polarized range; betting for value with an Ace, slow-playing a monster or he has absolutely nothing. Let’s look at the expect value of this situation.
Ev Formula Poker
Pot Odds: $24 in the pot / $8 to call = 3-to-1. You need to be correct at least 1 out of every 4 times. This means you need 25% equity versus his likely range of hands to be profitable in this situation.
50.342% { }
49.658% { KK+,22,A2s+,QTs-Q9s, T7s+, 96s+, 85s+, 74s+, 64s+, 54s, A2o+, QTo-Q9o, J9o-J8o, T8o+, 97o+, 87o.
These equity figures were calculated using Poker Stove – a very handy piece of software that will help you to analyze your hands and determine the maximum EV of each situation.
I included a wide variety of suited connectors, connectors, one gapped cards and hands he could have c-bet bluffed with on the flop, gave up on the turn, then bet the river because we didn’t act on the turn. Even if you remove some of the bluffing hands from his range it’s much more than the 25% you need. Therefore this call — clearly has expected value.
Hand Example – Blind vs. Blind
This next hand example is a blind vs. blind confrontation from a no-limit hold’em tournament. You have been playing fairly tight and have not made any recent moves. You are in the small blind. The big blind seems to be a fairly tight player, but also understands short stack dynamics.
Figure 3
Should you fold, call or shove? To simplify the example, let’s assume calling is not an option (since you are short stacked, it’s not an option anyway). To answer this, we need to assign a calling range to the big blind. Based on our read, it is fair to assume they would call with pocket pairs of 77+ and A7+. This comprises about 12% of all possible hand combinations. So we need to know how well we fare against his range if we do get called. To calculate this, we’ll use Poker Stove:
Obviously we are behind here, but our opponent is only calling with about 12% of all hands. This means that the BB folds 88% of the time. If we do get called and win, our stack will double to $4,000, and of course we’ll be at $0 if we lose.
Based on these calculations, here are all the possible outcomes:
- We fold: after posting the SB, our stack is now 1,850.
- We shove, BB folds: stack is 2,300 x 88% likelihood = $2024 expectation.
- We shove, BB calls, we win (12% calling X 31.275% winning = Occurs 3.753%): 4,000 x 3.753% = $150.12 expectation.
- We shove, BB calls, we lose (12% calling x 68.725% = Occurs 8.247%): $0 expectation.
If you add up all the possible outcomes for shoving, you get $2024 + $150.12 + $0 = $2174.12.
+2174.12: Total expectation of shoving
– 1850.00: Total expectation of folding
$324.12: Total expectation
As you can see, you gain an average of +$324.12 EV in the long run if you push all-in. But we just used a hand that was statistically better than an average hand the BB could hold. What if we pushed all-in with a trash hand, like 72os?
24.931% { }
75.069% { 77+, A7s+, A7o+ }
- We shove, BB folds: our stack is 2,300 x 88% = $2024 expectation.
- We shove, BB calls, we win (12% calling X 24.931% winning = Occurs 2.99%): 4,000 x 2.99% = $119.60 expectation
- We shove, BB calls, we lose (12% calling x 75.069% = Occurs 9.01%): $0 Expectation
+2143.60: Total expectation of shoving (2024 + 119.60)
– 1850.00: Total expectation of folding
Ev Formula Poker Expert
$293.60: Total expectation
So because our opponent is only calling with 12% of hands, our shove with the worst hand in poker is still highly profitable. You can play around with Poker Stove and change your opponent’s possible calling range to determine various expectations.
Hand Example – Hero Call on the River
This final example is also from a no-limit hold’em tournament, but this time we’re going to look at the play post-flop.
From the button, you raised pre-flop to 500 with and the big blind defended. On a flop of your opponent checks and you bet 850 into a pot of 1350:
Figure 4
The big blind (player 7) has shown to be a loose player capable of calling people down with marginal hands and chasing draws. He has also played his bigger hands strongly.
Your opponent calls your 850 bet. Now let’s look at hand ranges. Since your opponent is loose and likes to see the flop, his defending range pre-flop is pretty wide. Since he would most likely re-raise with AQ+ and 88+, I would assign a range of A2-AJ, K9+, Q9+, 22-77 and all connectors and one-gappers 56 – JT. Since he has called our continuation bet, we can narrow down his range. Based on our read of him playing bigger hands strong, we can eliminate AT, AJ, 66, 77, 67, A6 and A7. Removing those and all other hands that did not connect with this flop, we are down to A2-A5, A8,A9, 89, 56, 57, 97, 87, 86 and all flush draw combos. It’s possible he may have check-raised with a strong draw, but our read on this player is that he usually calls in these situations.
The Turn & River
The turn card brings a . Based on our read, this won’t change much unless our opponent turned two-pair with A2 or hit a set of 2’s. Our opponent checks and we check behind for pot control. The river is and our opponent shoves all-in for 3,625:
Figure 5
The pot is now 3,050 and our stack is also 3,625. The King is a tricky card because we are losing to any Ace and any suited King that was chasing a draw, but we are way ahead if our opponent was on a draw or had middle pair. Our opponent could have two-pair or a set, but based on our original read of his range this isn’t likely, however we cannot rule it out completely. So let’s look at the EV of the situation.
Pot Odds: 3050 in the pot + 3625 in his stack = 6675 and it’s 3625 to call. This is 6675/3625 or 1.83 to 1. So we cannot be behind more than 1.84-1 to make this call profitable.
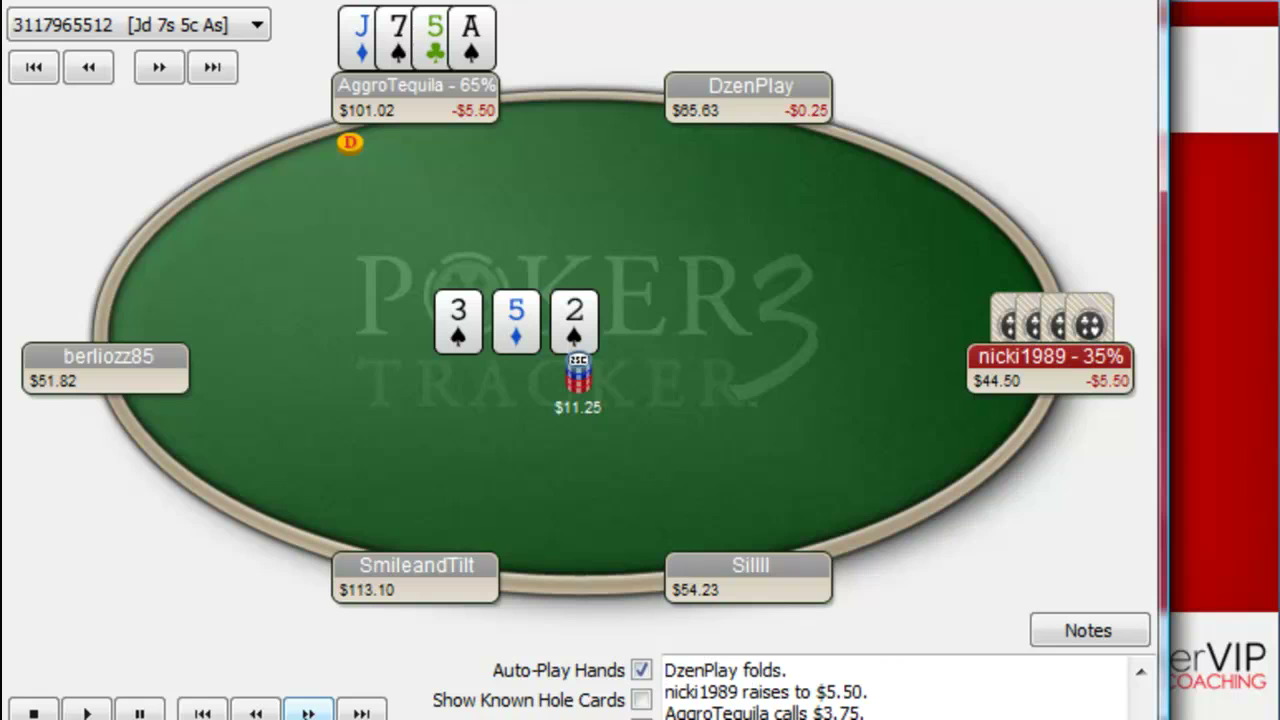
53.125% { }
46.875% { 55-22, A9s-A8s, A5s-A2s, KsQs, KsJs, KsTs, Ks9s, QsJs, QsTs, Qs9s, JsTs, Js9s, Ts9s, Ts8s, 9s8s, 9s7s, 8s7s, 8s6s, 75s, 65s, A9o-A8o, A5o-A2o, 97o+, 86o+, 75o, 65o }
Using Poker Stove, you can see that we are actually slightly ahead by 1.13 to 1. Simply based on pot odds, this would be a +EV call to make. But sometimes we need more confidence in iffy situations. Let’s see what our actual expected value is.
To calculate our expected value, here are all the possible outcomes:
- We fold: Stack is 3,625
- We call and win (53.125% of time) = 10,300 x 53.125% = $5471.87 expectation
- We call and lose (46.875% of time): $0 Expectation
+5,471.87: Total expectation of calling
– 3,625.00: Total expectation of folding
Poker Ev Formula With Fold Equity
$1,846.87: Total expectation
As you can see, by making this call you will win an average of $1,846.87 over time, giving it a high positive expectation.
Understanding ICM (Independent Chip Model)
In this lesson we’ve looked at a cash game hand and a couple of tournament examples. It’s important to point out that in cash games the expected value of your chips (cEV) equals the same amount as the real dollar value associated with them ($EV). This isn’t always the case when it comes to tournaments. In the beginning parts and middle stages of most tournaments cEV roughly equals $EV, however depending on the payout structure and how many players are remaining, there are times in which a decision can be +cEV but -$EV. An example of this would be on the bubble stage. ICM helps you determine your $EV in these situations. Please see our lesson on the independent chip model to learn more about this concept, which is vital if you wish to be a winning SNG / tournament player.
Conclusion
The best way to train yourself to consistently make +EV plays is to analyze key hands after each session of play. This will allow you to assign hand ranges with a clear head and make an unemotional assessment as to whether a play had positive or negative expectation. The more you train yourself to become analytical with these decisions, the more profit you will make at the poker tables.
Related Lessons
Ev Calculator Poker
By Donovan Panone
Donovan started playing poker in 2004 and is an experienced tournament and cash game player who has a passion for teaching and helping others improve their game.